Viscosity&s.g
Viscosity
Viscosity is an indication of how easily a fluid flows. When a fluid flows, the interaction between the molecules within the fluid creates resistance, which is shear stress.
The relationship viscosity and shear stress for Newtonian fluids are express as:
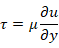
The relationship viscosity and shear stress for Newtonian fluids are express as:
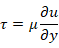
where,
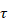= sheer stress
μ = dynamic viscosity of the flow
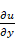= velocity gradient
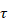= sheer stressμ = dynamic viscosity of the flow
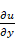= velocity gradient
Under the same flow conditions (identical velocity gradient), fluids with a higher viscosity will experience a higher shear stress.
High viscosity fluids cause additional pressure loss when flowing through a centrifugal pump, resulting in reduced head, increased shaft load, and reduced efficiency. Furthermore, high viscosity fluids will also result in greater piping loss, increasing the chance of cavitation at the pump suction. Commonly used units for viscosity are Pa-s and cP (centipoise), where 1 mPa-s = 1 cP. The viscosity for water under ambient temperature is 1 cP.
High viscosity fluids cause additional pressure loss when flowing through a centrifugal pump, resulting in reduced head, increased shaft load, and reduced efficiency. Furthermore, high viscosity fluids will also result in greater piping loss, increasing the chance of cavitation at the pump suction. Commonly used units for viscosity are Pa-s and cP (centipoise), where 1 mPa-s = 1 cP. The viscosity for water under ambient temperature is 1 cP.
Specific Gravity (S.G.)
Specific Gravity is the ratio of the density of a substance relative to the density of water, therefore, it is a dimensionless unit.
The S.G. of a fluid will be different under different concentration and under different temperature. For regular fluids, the higher the concentration of the fluid, the higher the Specific Gravity, and the higher the temperature, the lower the Specific Gravity.
Specific Gravity is a very important factor in pump selection. Pressure generated by the pump is directly proportional to the S.G. of the liquid.
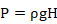Where,
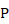= Pressure
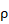= density of the liquid
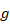= acceleration due to gravity
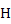= head
The pump’s hydraulic power is directly proportional to the S.G. of the liquid.
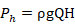Where,
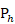= hydraulic power
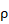= density of the liquid
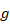= acceleration due to gravity
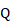= flow capacity
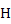= head
Therefore, from the above two relationships, we can also conclude that the Shaft power is also proportional to the S.G. of the liquid.
The S.G. of a fluid will be different under different concentration and under different temperature. For regular fluids, the higher the concentration of the fluid, the higher the Specific Gravity, and the higher the temperature, the lower the Specific Gravity.
Specific Gravity is a very important factor in pump selection. Pressure generated by the pump is directly proportional to the S.G. of the liquid.
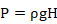
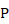= Pressure 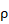= density of the liquid 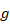= acceleration due to gravity 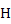= headThe pump’s hydraulic power is directly proportional to the S.G. of the liquid.
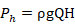
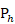= hydraulic power 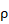= density of the liquid 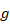= acceleration due to gravity 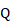= flow capacity 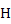= headTherefore, from the above two relationships, we can also conclude that the Shaft power is also proportional to the S.G. of the liquid.